Power is the rate of energy transfer or the rate at which work is done. In electrical engineering, understanding power and its related formulas is crucial in analyzing, designing, and troubleshooting electrical circuits. In this article, we will explore the power formulas used in DC and AC single-phase and three-phase circuits.

Introduction

Electric power is the product of voltage and current, measured in watts. Power is a fundamental parameter that needs to be accurately measured, controlled, and distributed in electrical systems. Power can be categorized into several types, such as apparent power, active power, and reactive power. In this article, we will discuss the power formulas used in DC and AC single-phase and three-phase circuits.

Power Formulas in DC and AC 1-Phase & 3-Phase Circuits | Complex Power Formulas

Average Power Formula in AC Circuit

In an AC (alternating current) circuit, the average power ( $P_{avg}$ ) delivered to a load can be calculated using the following formula:

P_{avg} = V_{rms} \times I_{rms} \times \cos(\phi)

Where:

$V_{rms}$ is the root mean square (RMS) value of the voltage.
$I_{rms}$ is the root mean square (RMS) value of the current.
$\cos(\phi)$ is the power factor of the circuit, with $\phi$ being the phase angle between the voltage and current.

The power factor $\cos(\phi)$ accounts for the phase difference between the voltage and current in the circuit. In purely resistive circuits, $\phi = 0$ and $\cos(\phi) = 1$ , so the average power is simply $P_{avg} = V_{rms} \times I_{rms}$ . In circuits with inductive or capacitive elements, the phase angle $\phi$ will not be zero, and $\cos(\phi)$ will be less than 1, reducing the average power delivered to the load.

Let's go through an example of calculating the average power in an AC circuit.

Example:

Consider an AC circuit where:

The RMS voltage $V_{rms} = 120 \, \text{V}$
The RMS current $I_{rms} = 10 \, \text{A}$
The phase angle $\phi = 30^\circ$

Step 1: Calculate the power factor

The power factor is given by $\cos(\phi)$

$\cos(30^\circ) = \frac{\sqrt{3}}{2} \approx 0.866$

Step 2: Calculate the average power

Use the formula for average power:

$P_{avg} = V_{rms} \times I_{rms} \times \cos(\phi)$

Substituting the given values:

$P_{avg} = 120 \, \text{V} \times 10 \, \text{A} \times 0.866 \approx 1039.2 \, \text{W}$

Final Answer:

The average power delivered to the load in this AC circuit is approximately 1039.2 watts.

Power in DC circuits

In DC circuits, power is calculated using the following formulas:

2.1 Power formulas

• Power (P) = Voltage (V) x Current (I)

• Power (P) = Current (I)^2 x Resistance (R)

• Power (P) = Voltage (V)^2 / Resistance (R) Power Calculator

Power Calculator

Enter the values for Voltage, Current, and Resistance to calculate Power using one of the following formulas:

Example

Power (P) = Voltage (V) x Current (I)

The above formula is used to calculate the power in a DC circuit when the voltage and current are known. For example, if a circuit has a voltage of 12 volts and a current of 2 amps, the power can be calculated as P = 12V x 2A = 24 watts.

Example

• Power (P) = (Current (I))^2 x Resistance (R)

The above formula is used to calculate the power in a DC circuit when the current and resistance are known. For example, if a circuit has a current of 3 amps and a resistance of 4 ohms, the power can be calculated as P = (3A)^2 x 4Ω = 36 watts.

Power measurement

The power in a DC circuit can be measured using a wattmeter, which is an instrument that measures the product of voltage and current.

2.3 Power efficiency

Power efficiency is the ratio of the output power to the input power. In a DC circuit, power efficiency can be calculated as follows:

• Efficiency = Output power / Input power

3. Power in AC single-phase circuits

In AC single-phase circuits, power can be divided into three types: apparent power, active power, and reactive power.

3.1 Apparent power

Apparent power is the product of the voltage and current, measured in VA (volt-amperes). It represents the total power consumed by a circuit, including both the active power and the reactive power. Apparent power can be calculated as follows:

• Apparent power (S) = Voltage (V) x Current (I)

3.2 Active power

Active power is the real power that is consumed by the circuit, measured in watts. Active power can be calculated as follows:

• Active power (P) = Voltage (V) x Current (I) x Power factor (PF)

3.3 Reactive power

Reactive power is the imaginary power that is stored and released by reactive components in the circuit, such as inductors and capacitors. Reactive power is measured in vars (volt-amperes reactive) or kVAR (kilovolt-amperes reactive). Reactive power can be calculated as follows:

• Reactive power (Q) = √(Apparent power^2 - Active power^2)

Power in three-phase circuits

In three-phase circuits, power is calculated using the line and phase voltages and currents. Three-phase systems can deliver more power with less voltage drop compared to single-phase systems.

4.1 Line and phase voltages and currents

In three-phase circuits, the voltage and current can be measured in two ways: line-to-line (LL) and line-to-neutral (LN) voltages and currents. The line-to-line voltage is the voltage between two phases, while the line-to-neutral voltage is the voltage between one phase and the neutral wire. The line-to-line current is the current flowing through two phases, while the line-to-neutral current is the current flowing through one phase.

4.2 Apparent, active, and reactive power in three-phase systems

In three-phase systems, power can be calculated using the following formulas:

• Apparent power (S) = √3 x Voltage (VL-L) x Current (IL-L)

• Active power (P) = √3 x Voltage (VL-L) x Current (IL-L) x Power factor (PF)

• Reactive power (Q) = √(Apparent power^2 - Active power^2)

Power factor

Power factor is a measure of the efficiency of the electrical system, defined as the ratio of the active power to the apparent power. It represents the degree to which the electrical system is utilizing the available power. A high power factor indicates that the electrical system is using power efficiently, while a low power factor indicates that the electrical system is not using power efficiently.

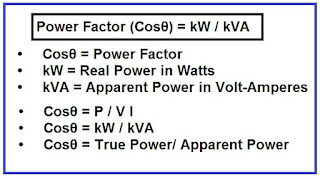
Power Factor (Cosθ) = kW / kVA

Where
• Cosθ = Power Factor
• kW = Real Power in Watts
• kVA = Apparent Power in Volt-Amperes or Watts
Formulas used for power factor.
• Cosθ = P / V I
• Cosθ = kW / kVA
• Cosθ = True Power/ Apparent Power

Power Formulas in Complex Circuits

In complex AC circuits, the power factor is often less than 1, which means that the voltage and current waveforms are not in phase with each other. In such cases, the apparent power (S) is not equal to the true power (P). To calculate the true power in these circuits, we use complex power and power factor.

The complex power (S) is the vector sum of the real power (P) and the reactive power (Q), and is given by the formula:

S = P + jQ

where j is the imaginary unit. The real power (P) is the power dissipated in the circuit, while the reactive power (Q) is the power stored and returned to the source in each cycle.

The apparent power (S) can be calculated using the formula:

S = Vrms x Irms

where Vrms is the root mean square (RMS) voltage and Irms is the RMS current.

The power factor (PF) is the ratio of the real power (P) to the apparent power (S), and is given by the formula:

PF = P/S

Using these formulas, we can calculate the true power (P) in complex AC circuits as:

P = S x PF

We can also calculate the reactive power (Q) as:

Q = S x √(1 - PF^2)

where √ is the square root function.

Conclusion

In conclusion, power is a fundamental parameter in electrical engineering, and understanding the power formulas is crucial in analyzing, designing, and troubleshooting electrical circuits. We have discussed the power formulas used in DC and AC single-phase and three-phase circuits, including the formulas for apparent power, active power, and reactive power. We have also discussed power factor, which is an important measure of the efficiency of the electrical system.

FAQs

1. What is power in electrical engineering?

• Power is the rate of energy transfer or the rate at which work is done, measured in watts.

2. What is the formula for calculating power in a single-phase AC circuit?

• Power in a single-phase AC circuit can be calculated using the formula P = V x I x cos(θ), where P is the power in watts, V is the voltage in volts, I is the current in amperes, and cos(θ) is the power factor.

3. What is the formula for calculating power in a three-phase AC circuit?

• The formula for calculating power in a three-phase AC circuit is P = √3 x V x I x cos(θ), where P is the power in watts, V is the line-to-line voltage in volts, I is the line current in amperes, and cos(θ) is the power factor.

4. What are the three formulas for power?

• The three formulas for power are P = V x I, P = I^2 x R, and P = V^2 / R, where P is the power in watts, V is the voltage in volts, I is the current in amperes, and R is the resistance in ohms.

5. What is the formula for power in a DC circuit?

• The formula for power in a DC circuit is P = V x I, where P is the power in watts, V is the voltage in volts, and I is the current in amperes.

Electrical Technology Solutions - Advancement

Power Formulas in DC and AC 1-Phase & 3-Phase Circuits | Average Power Formula | Complex Power Formulas | Reactive Power Formula | Power Factor Formula | Electrical Power Formula | Power Formula | Average Power Formula in AC Circuit

Introduction